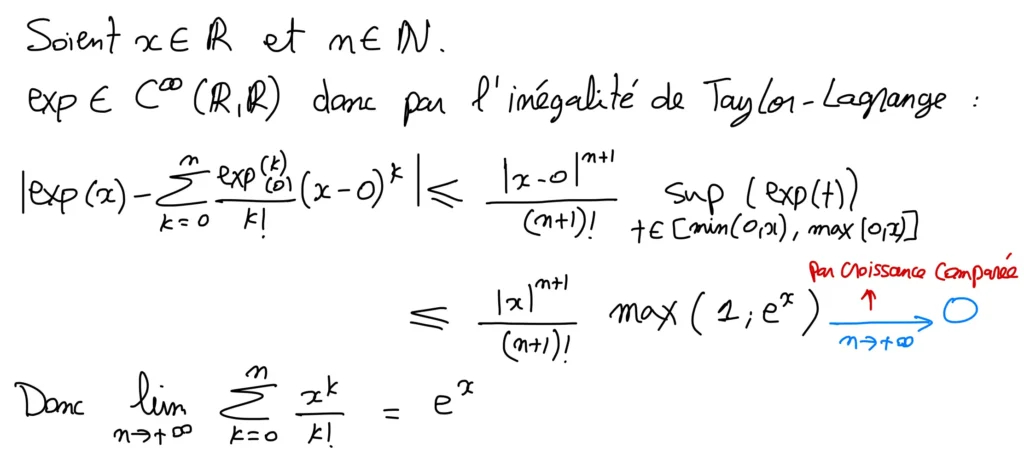Ce cours sur l’intégration est conforme au programme des filières MPSI et MP2I. Il contient des exemples et des exercices d’application qui faciliteront votre assimilation du contenu. N’hésitez pas à consulter notre série d’exercices corrigés pour acquérir une maîtrise solide de cours.

Cours d’intégration programme MPSI et MP2I

Dans tout ce cours \mathbb{K} désigne \mathbb{R} ou \mathbb{C} , a, b deux réels tels que a<b.

I) Continuité uniforme

Définition 1 :
Soient I un intervalle de \mathbb{R} et f \ : \ I \rightarrow \mathbb{K} une fonction.
On dit que f est uniformément continue sur I lorsque :
\forall \varepsilon \gt 0, \ \exists \eta \gt 0, \ \forall x,y \in I, \ (|x-y| \leq \eta \Rightarrow |f(x)-f(y)| \leq \varepsilon )

Remarque 1 :
i) Toute fonction lipschitzienne sur I est uniformément continue sur I .
ii) Toute fonction uniformément continue sur I est continue sur I .
iii) Les réciproques de i) et ii) sont fausses.

Théorème de Heine :
Toute fonction continue sur un segment y est uniformément continue.

II) Fonctions continues par morceaux

1) Subdivision d’un segment de \mathbb{R}

Définition 1 (Subdivision) :
On appelle subdivision du segment [a, b] toute famille finie de nombres réels \sigma=\left(x_{k}\right)_{0 \leq k \leq n} tels que : a=x_{0}<x_{1}<\cdots<x_{n-1}<x_{n}=b.
Le pas de la subdivision \sigma est le réel : \delta(\sigma)=\max _{i \in \llbracket 0, n-1 \rrbracket}\left(x_{i+1}-x_{i}\right).
La subdivision est dite régulière lorsque pour tout i \in \llbracket 0, n-1 \rrbracket , \ \ x_{i+1}-x_{i}=\delta(\sigma).
Le support de le subdivision \sigma est l’ensemble \left\{x_{i} / i \in \llbracket 0, n-1 \rrbracket\right\}, on le note \operatorname{Supp}(\sigma).
Si \sigma^{\prime} est une autre subdivision de [a, b], on note \sigma \cup \sigma^{\prime} la subdivision de [a, b] dont le support est \operatorname{Supp}(\sigma) \cup \operatorname{Supp}\left(\sigma^{\prime}\right).

Exemple 1 :
i) On pose x_{0}=0, \ x_{1}=2,\ x_{2}=4, \ x_{3}=6,\ x_{4}=8, \ x_{5}=10 .
\sigma=\left(x_{k}\right)_{0 \leq k \leq 5} est une subdivision régulière de [0,10] de pas \delta(\sigma)=2 et de support  \operatorname{Supp}(\sigma)=\{0 ; 2 ; 4 ; 6 ; 8 ; 10\}
ii) On pose y_{0}=0, \ y_{1}=3,\ y_{2}=10. \sigma^{\prime}=\left(y_{k}\right)_{0 \leq k \leq 5} est une subdivision non régulière de [0,10] de pas \delta\left(\sigma^{\prime}\right)=7 et de support \operatorname{Supp}\left(\sigma^{\prime}\right)=\{0 ; 3 ; 10\}.
iii) On pose z_{0}=0,\ z_{1}=2, \ z_{2}=3,\ z_{3}=4, \ z_{4}=6,\ z_{5}=8, \ z_{6}=10. On a \sigma \cup \sigma^{\prime}=\left(z_{k}\right)_{0 \leq k \leq 6}.
iv) On pose pour tout k \in \llbracket 0, n \rrbracket, a_{k}=a+k \frac{b-a}{n} .
\tau=\left(a_{k}\right)_{0 \leq k \leq n} est une subdivision régulière de [a, b] de pas \delta(\tau)=\frac{b-a}{n} et de support \operatorname{Supp}(\tau)=\left\{a+k \frac{b-a}{n} / k \in \llbracket 0, n \rrbracket\right\}.

Définition 2 (Subdivision plus fine qu’une autre) :
Soient \sigma et \tau deux subdivision du segment [a, b].
On dit que \tau est plus fine que \sigma lorsque \operatorname{Supp}(\sigma) \subset \operatorname{Supp}(\tau).

Exemple 2 :
i) On pose x_{0}=0, \ x_{1}=2,\ x_{2}=4,\ x_{3}=6,\ x_{4}=8, \ x_{5}=10 et y_{0}=0,\ y_{1}=4,\ y_{2}=8, \y_{3}=10.
La subdivision \sigma=\left(x_{k}\right)_{0 \leq k \leq 5} est plus fine que la subdivision \sigma^{\prime}=\left(y_{k}\right)_{0 \leq k \leq 3}.
ii) Si \sigma et \sigma^{\prime} sont deux subdivisions de [a, b] alors \sigma \cup \sigma^{\prime} est une subdivision du segment [a, b] plus fine que \sigma et que \sigma^{\prime}.

2) Fonctions en escaliers

Définition 3 (Fonction en escalier sur un segment de \mathbb{R} ) :
Soit \varphi \in \mathcal{F}([a, b], \mathbb{K}), on dit que \varphi est une fonction en escalier sur le segment [a, b] lorsqu’il existe une subdivision \sigma=\left(x_{k}\right)_{0 \leq k \leq n} de [a, b] telle que \varphi est constante sur chaque intervalle ] x_{k}, x_{k+1}[.
C’est-à-dire : \left.\forall k \in \llbracket 0, n-1 \rrbracket, \exists c_{k} \in \mathbb{K}, \forall x \in\right] x_{k}, x_{k+1}\left[, \varphi(x)=c_{k}\right.
On dit dans ce cas que la subdivision \sigma est adaptée à la fonction en escalier \varphi.
On note \mathcal{E}([a, b], \mathbb{K}) l’ensemble des fonctions en escalier sur [a, b] à valeurs dans \mathbb{K}.

Exemple 3 :
i) Si f est une fonction constante sur le segment [a, b] alors f est une fonction en escalier sur [a, b] et toute subdivision du segment [a, b] est adaptée à f.
ii) La restriction, au segment [a, b], de la fonction partie entière est une fonction en escalier sur [a, b] et la subdivision de support ([a, b] \cap \mathbb{N}) \cup\{a ; b\} est adaptée à f.
iii) La fonction f définie pour tout x \in[0,1] par f(x)= \begin{cases} -1, \ si \ x \in [0, \frac{1}{2} ] \\ 1, \ si \ x \in ]\frac{1}{2} , 1]\end{cases} est une fonction en escalier sur [0,1].
On pose x_{0}=0, \ x_{1}=\frac{1}{2}, \ x_{2}=1. La subdivision \sigma=\left(x_{k}\right)_{0 \leq k \leq 2} est adaptée à f.

Remarque 1 :
Soit f \in \mathcal{E}([a, b], \mathbb{K}) et \sigma une subdivision de [a, b] adaptée à f.
Si \tau est une subdivision de [a, b] plus fine que \sigma alors \tau est une subdivision adaptée à f.

Proposition 1 :
Soient f, g \in \mathcal{E}([a, b], \mathbb{K}) et \lambda \in \mathbb{K}.
i) \lambda f+g \in \mathcal{E}([a, b], \mathbb{K}). En particulier (\mathcal{E}([a, b], \mathbb{K}),+, \cdot) est un \mathbb{K}-espace vectoriel.
ii) f \times g \in \mathcal{E}([a, b], \mathbb{K})
iii) |f| \in \mathcal{E}([a, b], \mathbb{K})

Démonstration :
Soient \sigma , \sigma{'} deux subdivisions de [a, b] adaptées respectivement aux fonctions en escalier f \ et \ g.
Il est clair que les fonctions \lambda f +g, \ fg, \ |f| sont des fonctions en escalier car \sigma \cup \sigma{'} est une subdivision de [a, b] adaptée à ces trois fonctions.

Remarque 2 :
Une fonction en escalier sur un segment prend un nombre finie de valeurs, elle est donc bornée.

3) Fonctions continues par morceaux.

Définition 4 (Fonction continue par morceaux sur un segment de \mathbb{R} ) :
Soit f \in \mathcal{F}([a, b], \mathbb{K}), on dit que f est une fonction continue par morceaux sur le segment [a, b] lorsqu’il existe une subdivision \sigma=\left(x_{k}\right)_{0 \leq k \leq n} de [a, b] telle que \forall k \in \llbracket 0 ; n \rrbracket, f_{/] x_{k}, x_{k+1}[} est continue sur l’intervalle ]x_{k}, x_{k+1} [ et admet des limites finies à droite en x_{k} et à gauche en x_{k+1}.
On dit dans ce cas que la subdivision \sigma est adaptée à la fonction f.
On note C M([a, b], \mathbb{K}) l’ensemble des fonctions continues par morceaux sur [a, b] à valeurs dans \mathbb{K}.

Exemple 4 :
i) Toute fonction continue sur un segment y est continue par morceaux.
ii) Toute fonction en escalier sur un segment y est continue par morceaux.
iii) La fonction f définie pour tout x \in[0,1] par f(x)= \begin{cases}-2, \ si \ x=0 \\ x-1, \ si \ x \in ] 0, \frac{1}{3} ] \\ \frac{1}{x}, \ si \ x \in ] \frac{1}{3}, 1[ \\ 5, \ si \ x=1 \end{cases} est une fonction continue par morceaux sur [0,1].
On pose x_{0}=0, \ x_{1}=\frac{1}{3}, \ x_{2}=1. La subdivision \sigma=\left(x_{k}\right)_{0 \leq k \leq 2} est adaptée à f.

Remarque 3 :
Soient f \in C M([a, b], \mathbb{K}) et \sigma une subdivision de [a, b] adaptée à f.
Si \tau est une subdivision de [a, b] plus fine que \sigma alors \tau est une subdivision de [a, b] adaptée à f.

Proposition 2 :
Soient f, g \in C M([a, b], \mathbb{K}) et \lambda \in \mathbb{K}.
i) \lambda f+g \in C M([a, b], \mathbb{K}). En particulier (C M([a, b], \mathbb{K}),+, \cdot) est un \mathbb{K}-espace vectoriel.
ii) f \times g \in C M([a, b], \mathbb{K}).
iii) |f| \in C M([a, b], \mathbb{K}).

Démonstration :
Soient \sigma , \sigma{'} deux subdivisions de [a, b] adaptées respectivement aux fonctions continues par morceaux f \ et \ g.
Il est clair que les fonctions \lambda f +g, \ fg, \ |f| sont des fonctions continues par morceaux car \sigma \cup \sigma{'} est une subdivision de [a, b] adaptée à ces trois fonctions.

Proposition 3 :
Toute fonction continue par morceaux sur un segment de \mathbb{R} y est bornée. (Mais n’atteint pas forcément ses bornes).

Démonstration :

III) Intégration d’une fonction continue par morceaux sur un segment

1) Intégrale d’une fonction en escalier

Proposition-définition 1 (Intégrale d’une fonction en escalier) :
Soient \varphi \in \mathcal{E}([a, b], \mathbb{R}) et \sigma=\left(x_{k}\right)_{0 \leq k \leq n} une subdivision adaptée à \varphi.
\left.\forall k \in \llbracket 0, n-1 \rrbracket, \exists c_{k} \in \mathbb{R}, \forall x \in\right] x_{k}, x_{k+1}\left[, \varphi(x)=c_{k}\right..
On note I_{\varphi, \sigma}=\sum_{k=0}^{n-1} c_{k}\left(x_{k+1}-x_{k}\right).
Ce nombre réel ne dépend pas de la subdivision \sigma choisie, on l’appelle intégrale de la fonction \varphi \operatorname{sur}[a, b].
On le note : \int_{a}^{b} f(t) d t, \int_{a}^{b} f, ou \int_{[a, b]} f.

Démonstration :

Exemple 5 :
i) Si f est constante égale à c alors \int_{a}^{b} f(x) d x=c(b-a). Car la famille (a, b) est une subdivision de [a, b] adaptée à f.
ii) \forall n \in \mathbb{N}^{*}, \int_{0}^{n}\lfloor x\rfloor d x=\sum_{k=0}^{n-1} k((k+1)-k)=\frac{n(n-1)}{2}.
En effet, pour tout entier naturel non nul n, la famille (k)_{k \in \llbracket 0, n \rrbracket} est une subdivision du segment [0, n] adaptée à la fonction en escalier x \rightarrow\lfloor x\rfloor et \forall x \in] k, k+1[,\lfloor x\rfloor=k.

Proposition 4 (Propriétés de l’intégrale des fonctions en escalier) :
Soient f, g \in(\mathcal{E}([a, b], \mathbb{R}),+, \cdot) et \lambda \in \mathbb{R}. Alors :
i) Linéarité de l’intégrale : \int_{a}^{b}(\lambda f(x)+g(x)) d x=\lambda \int_{a}^{b} f(x) d x+\int_{a}^{b} g(x) d x.
ii) Positivité de l’intégrale : Si f \geq 0, alors \int_{a}^{b} f(x) d x \geq 0.
iii) Croissance de l’intégrale : Si f \leq g, alors \int_{a}^{b} f(x) d x \leq \int_{a}^{b} g(x) d x.
iv) Inégalité triangulaire intégrale : \left|\int_{a}^{b} f(x) d x\right| \leq \int_{a}^{b}|f(x)| d x.
v) Relation de Chasles : Pour tout c \in[a, b], \int_{a}^{b} f(x) d x=\int_{a}^{c} f(x) \mathrm{dx}+\int_{c}^{b} f(x) dx. En particulier \int_{b}^{a} f(x) d x=-\int_{a}^{b} f(x) d x.

Démonstration :

2) Approximation d’une fonction continue par morceaux par des fonctions en escaliers.

Proposition 5 :
Soit f \in C^{0}([a, b], \mathbb{R}).
\forall \varepsilon>0, \exists \varphi \in \mathcal{E}([a, b], \mathbb{R}), \forall x \in[a, b],|f(x)-\varphi(x)| \leq \varepsilon.

Démonstration :

Proposition 6 :
Soit f \in C M([a, b], \mathbb{R}).
\forall \varepsilon>0, \exists \varphi \in \mathcal{E}([a, b], \mathbb{R}), \forall x \in[a, b],|f(x)-\varphi(x)| \leq \varepsilon.

Démonstration :

Corollaire 1 :
Soient f \in C M([a, b], \mathbb{R}) et \left(u_{n}\right)_{n \in \mathbb{N}} une suite à valeurs dans \mathbb{R}^{*}_{+}qui converge vers 0 . On a :
\forall n \in \mathbb{N}, \exists \varphi_{n} \in \mathcal{E}([a, b], \mathbb{R}), \forall x \in[a, b], \ \ \left|f(x)-\varphi_{n}(x)\right| \leq u_{n}.

Démonstration :
Pour n \in \mathbb{N} on applique la proposition précédente avec \varepsilon = u_n .

3) Intégrale d’une fonction continue par morceaux

Proposition et définition 2 :
Soient f \in C M([a, b], \mathbb{R}), \ \left(u_{n}\right)_{n \in \mathbb{N}} une suite à valeurs dans \mathbb{R}^{*}_{+}qui converge vers 0 et \left(\varphi_{n}\right) \in \mathcal{E}([a, b], \mathbb{R})^{\mathbb{N}} tels que : \forall n \in \mathbb{N}, \forall x \in[a, b],\left|f(x)-\varphi_{n}(x)\right| \leq \mathrm{u}_{\mathrm{n}}. On a :
La suite \left(\int_{[a, b]} \varphi_{n}\right) est convergente et sa limite ne dépend ni de \left(u_{n}\right)_{n \in \mathbb{N}}, ni de \left(\varphi_{n}\right)_{n \in \mathbb{N} } . Cette limite est appelée intégrale de f sur [a, b], on la note \int_{a}^{b} f(x) dx .

Démonstration :

Proposition 7 :
Soient f, g \in C M([a, b], \mathbb{R}) et \lambda \in \mathbb{R}.
i) Linéarité de l’intégrale : \int_{a}^{b}(\lambda f(x)+g(x)) d x=\lambda \int_{a}^{b} f(x) d x+\int_{a}^{b} g(x) d x
ii) Positivité de l’intégrale : Si f \geq 0, alors \int_{a}^{b} f(x) d x \geq 0.
iii) Croissance de l’intégrale : Si f \leq g, alors \int_{a}^{b} f(x) d x \leq \int_{a}^{b} g(x) d x.
iv) Inégalité triangulaire intégrale : \left|\int_{a}^{b} f(x) d x\right| \leq \int_{a}^{b}|f(x)| d x.
v) Relation de Chasles : Pour tout c \in[a, b], \int_{a}^{b} f(x)=\int_{a}^{c} f(x)+\int_{c}^{b} f(x). En particulier \int_{b}^{a} f(x)=-\int_{a}^{b} f(x).

Démonstration :

Proposition 8 :
L’intégrale d’une fonction f continue par morceaux sur [a, b] ne change pas si on change un nombre fini de valeurs prises f.

Démonstration :

4) Intégration des fonctions à valeurs dans \mathbb{C}

Les propriétés des fonctions continues par morceaux à valeurs dans \mathbb{R} (à l’exception de celle en rapport avec l’ordre) sont valables pour les fonctions continues par morceaux à valeurs dans \mathbb{C}. Et pour f \in C M([a, b], \mathbb{C}) on a :
\int_{a}^{b} f(t) d t=\int_{a}^{b} \operatorname{Re}(f)(t) d t+i \int_{a}^{b} \operatorname{Im}(f)(t) d t.

IV) Lien entre intégrale et primitive

Théorème fondamentale de l’analyse :
Soient f une fonction continue sur un intervalle I et a \in I. On a les assertions suivantes :
i) La fonction x \rightarrow \int_{a}^{x} f(t) d t est une primitive de f sur I.
ii) La fonction x \rightarrow \int_{a}^{x} f(t) d t est l’unique primitive de f qui s’annule en a.
iii) Pour toute primitive G de f, on a : \forall x \in I, G(x)=G(a)+\int_{a}^{x} f(t) d t.

Démonstration :

Proposition 9 :
Soit f \in C^{0}([a, b], \mathbb{R}) telle que f \geq 0.
Si \int_{a}^{b} f(x) d x=0 alors f est identiquement nulle sur [a, b].

Démonstration :

Proposition 10 :
Soit f \in C^{0}([a, b], \mathbb{R}).
\exists c \in[a, b], \frac{1}{b-a} \int_{a}^{b} f(x) d x=f(c).
f(c) est appelée la valeur moyenne de f sur [a, b].,

Démonstration :

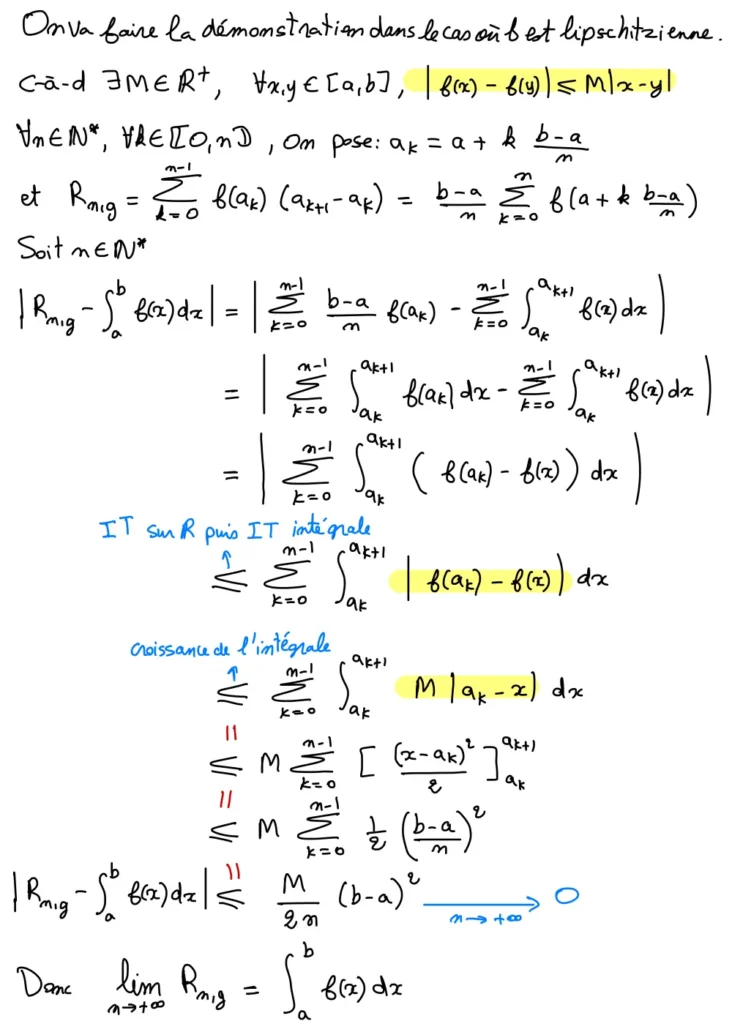
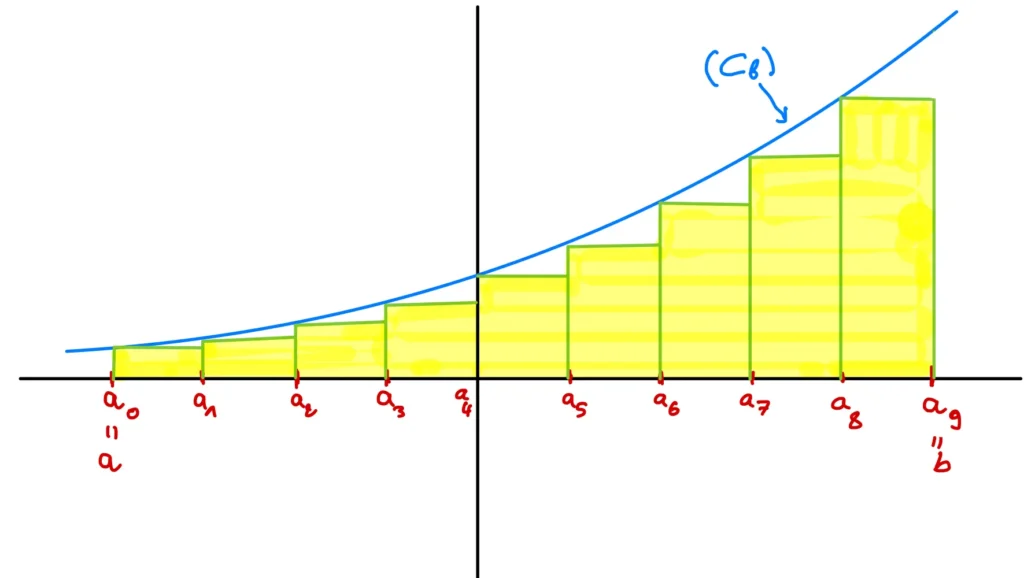
V) Sommes de Riemann

Convergence des sommes de Riemann :
Soit f \in C^{0}([a, b], \mathbb{R}). On a :
i) \frac{b-a}{n} \sum_{k=0}^{n-1} f\left(a+k \frac{b-a}{n}\right) \underset{n \rightarrow+\infty}{\rightarrow} \int_{a}^{b} f
ii) \frac{b-a}{n} \sum_{k=1}^{n} f\left(a+k \frac{b-a}{n}\right) \underset{n \rightarrow+\infty}{\rightarrow} \int_{a}^{b} f

Démonstration :

Interprétation géométrique :

La somme des aires des rectangles approche la valeur de l’intégrale de f de a à b

Exercice :
On pose pour tout n \in \mathbb{N}, u_{n}=\sum_{k=1}^{n} \frac{1}{k+n} et v_{n}=\sum_{k=0}^{2 n-1} \frac{k}{k^{2}+n^{2}}.
Déterminer les limites des suites \left(u_{n}\right) et \left(v_{n}\right).

Corrigé :
i) Soit n\in \mathbb{N^{*} } .
u_{n}=\sum_{k=1}^{n} \frac{1}{k+n} = \frac {1}{n} \sum_{k=1}^{n} \frac {1} { \frac{k}{n} +1} = \frac {1-0}{n} \sum_{k=1}^{n} f(0+k \frac {1-0}{n} ) avec f la fonction définie par : \forall x \in [0,1], \ f(x)= \frac{1}{x+1} .
Donc \underset{n \to +\infty }{\lim } u_{n} = \int_{0}^{1} f(x) dx = \int_{0}^{1} \frac{1}{x+1} dx = [ln(x+1]_{0}^{1} = \ln(2) .
ii) Soit n \in \mathbb{N^{*} } .
v_{n}=\sum_{k=0}^{2 n-1} \frac{k}{k^{2}+n^{2}} = \frac {2-0}{2n} \sum_{k=0}^{2n-1} \frac {\frac {2k}{2n} } { (\frac{2k}{2n})^2 +1} =\frac {2-0}{2n} \sum_{k=0}^{2n-1} f(0+k \frac{2-0}{2n}) .
avec f la fonction définie par : \forall x \in [0,2], \ f(x)= \frac{x}{x^{2}+1} .
Donc \underset{n \to +\infty }{\lim } v_{n} = \int_{0}^{2} f(x) dx = \int_{0}^{2} \frac{x}{x^{2}+1} dx = \frac{1}{2} [\ln (x^{2}+1) ]_{0}^{2} = \frac{\ln(5)}{2} .

VI) Formules de Taylor

Formule de Taylor avec reste intégral :
Soient f \in \mathcal{C}^{n+1}(I, \mathbb{R}) et a, x \in I. Alors
f(x)=\sum_{k=0}^{n} \frac{f^{(k)}(a)}{k !}(x-a)^{k}+\int_{a}^{x} \frac{f^{(n+1)}(t)}{n !}(x-t)^{n} d t.

Démonstration :

Remarque 4 :
A l’aide de la formule de Taylor avec reste intégral on démontre la formule de Taylor-Young.

Exemple 7 :
i) \forall x \in \mathbb{R}, \quad e^{x}=\sum_{k=0}^{n} \frac{x^{k}}{k !}+\int_{0}^{x} \frac{\mathrm{e}^{\mathrm{t}}}{n !}(x-t)^{n} d t
ii) \forall x \in \mathbb{R}, \quad \sin (x)=x-\frac{x^{3}}{6}+\int_{0}^{x} \frac{\sin (t)}{6}(x-t)^{3} d t.

Inégalité de Taylor-Lagrange :
Soient f \in \mathcal{C}^{n+1}(I, \mathbb{R}) et a, b \in I. Alors :
\left|f(b)-\sum_{k=0}^{n} \frac{f^{k}(a)}{k !}(b-a)^{k}\right| \leq \frac{|b-a|^{n+1}}{(n+1) !} \sup _{x \in[\min (a, b), \max (a, b)]}\left|f^{(n+1)}(x)\right|.

Démonstration :

Exemple 8 :
 \forall x \in \mathbb{R}, \ e^{x}=\sum_{k=0}^{+\infty} \frac{x^{k}}{k !}.

Corrigé :